Options
Gatcha math and the Featured 6* crystal (not a cry for help. probably)
So I finally ended my run on the current featured 6* crystal today, when I pulled the last thing I was hunting for (OG Scarlet Witch) on pull #69. This is by far the most featured crystals I've ever opened for one version, fueled by my ludicrous pursuit of Scarlet Witch and the fact that 6* shards are just a little too available in a little too many game modes for my own sanity.
Sixty nine crystals sounds like a lot, but it actually isn't a statistically significant amount if you are trying to, say, prove that every drop has an equal chance of falling. You'd need tens of thousands of crystals to do that. It is enough to demonstrate that most of the silly conspiracy theories are just that: silly. Here's my overall distribution of drops:
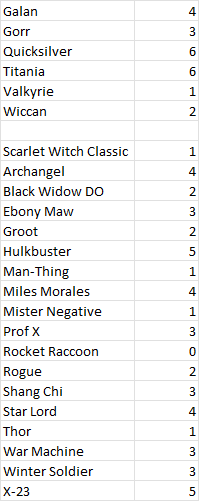
Out of 69 crystals, 22 were featured drops. The statistical average would be about 17 (69/4). So the crystal isn't significantly biased away from the new featured champs. There's not enough data to prove they are *exactly* balanced, but they are statistically unlikely to be biased low. If I should normally get 17 and I actually got 22, that might be lucky. But if someone thinks they are biased in such a way so that featureds come up, say, half as often as they should, then I got 22 when the crystals are rigged to generate something closer to 8. That's very, very unlikely.
69 crystals isn't enough to show that any one particular drop lands statistically at the presumptive percentage, but is it enough data to show *anything* statistically interesting? As it turns out, it is. We can analyze whether the crystals roughly follow gatcha math. What do I mean by that?
Gatcha mechanics generally refers to the situation where a person/player is confronted with an opportunity to get a random reward, selected from a set of rewards, where the presumptive desire is to collect the entire set (or some desirable subset of the set). Now, most people don't actually try to get all 24 champions in the featured crystal (if for no other reason than most people tend to have some of them already), but what if I analyze the data *as if* I was trying to collect them all. Is there enough data to see if the crystal's random drops roughly follows what I would expect? In at least rough terms, it is.
Let's answer one question first. If you were trying to get at least one of all twenty four champions from the crystal, about how long would that take? This isn't math that is often discussed. You might think you could calculate the odds of pulling the first champ, then the odds of pulling the second champ, and so on. But that doesn't work. While trying to pull the fifteenth champ, you might pull the eighteenth champ first, out of order. Then the math vastly overestimates how long it takes. But as it turns out, there is a relatively simple way to calculate this. We look at the average number of pulls it takes before you pull a champ you haven't pulled yet. If we go step by step, the answer drops out naturally.
We start with no champs and no crystals. How many crystals will it take before we pull a champ we haven't pulled yet? Obviously: 1. Whatever is in that crystal, it is guaranteed to be a new pull. Okay, we have one champion. Now how long will it take to pull a new champ? Well, 23 of the 24 champs would be new, and one would be a dup. If it were 12 new champs out of 24, the odds of pulling a new champ would be 50% - 12/24. And the number of crystals we would need to pull, on average, before we got a new champ would be 2, basically the reciprocal of the odds of a new champ. It is pretty obvious when the numbers are easy. But we generalize this for all situations: the odds of pulling a new champ are X/24, where X is 24 minus the number of new champs you have so far. And the average number of crystals you would need to open to get a new champ is the reciprocal of that, or 24/(1-new).
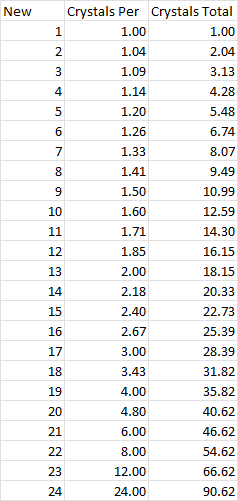
So to get one new champ it would take 24/24 crystals. To get two new champs would take 24/24 + 24/23 crystals. To get three new champs would take 24/24 + 24/23 + 24/22 crystals, and so on. We get this table:
Now these are all my drops:

And this is a table of how many new champs I had, alongside the statistical average expected:
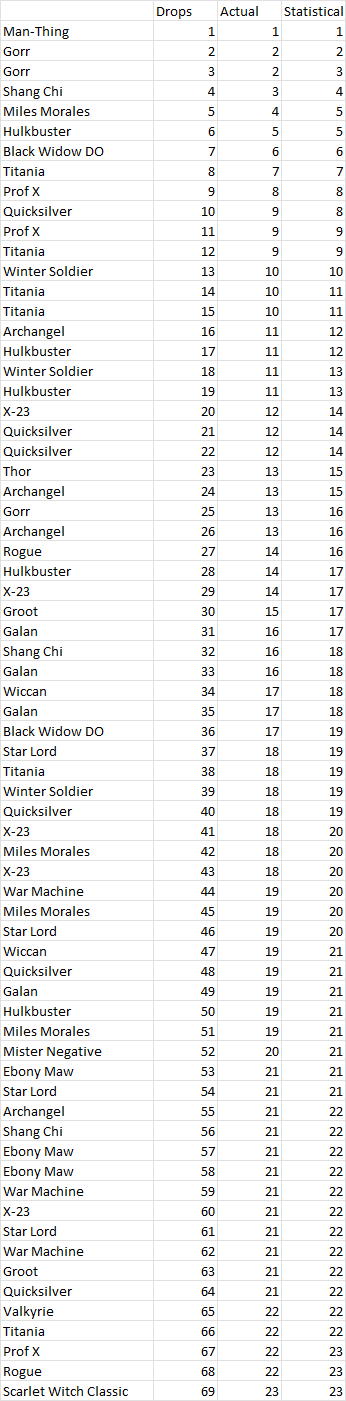
And how close was my distribution to the predicted gatcha new drop rate?
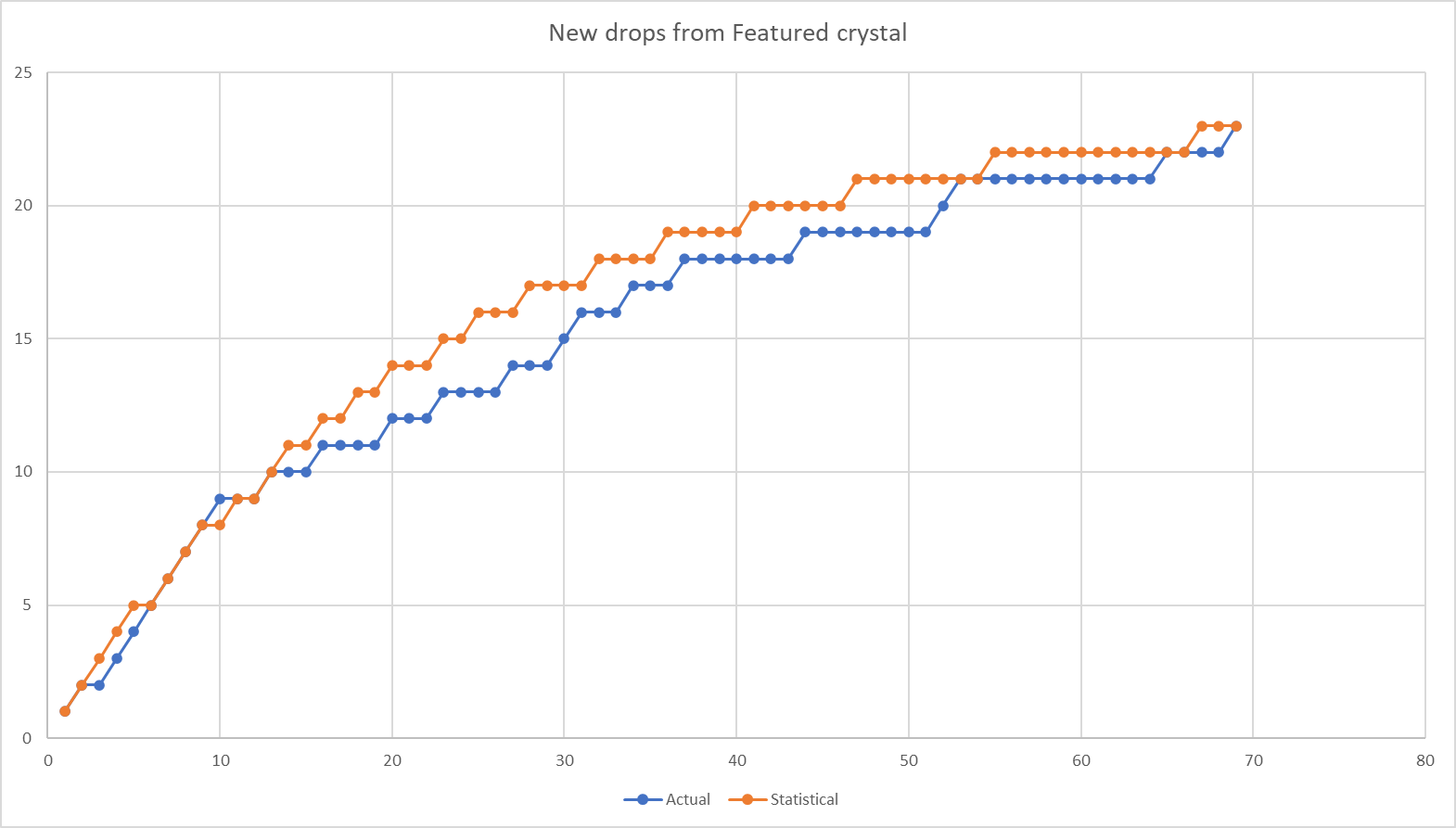
Pretty close. The crystal behaves, over long stretches, about as you would expect if each drop was roughly identical in drop rate. Now, this doesn't *prove* they are all identical in drop rates. It just shows they behave *like* they would. This means certain kinds of "rigging" are unlikely, in particular while it is statistically possible for some champs to be more likely than average, it is not statistically likely that some champs are far less likely than average. More likely champs would show up earlier, which would show up in the data as the blue line starting off higher than the orange line on the left side of the chart. But champs that were deliberately made much rarer would cause the blue line to drop progressively much lower than the orange line as you went to the right. That doesn't appear to be the case.
In other words, if Groot was twice as likely as every other champ, this sort of analysis wouldn't catch that (although the fact that I only pulled Groot twice would strongly contradict that conjecture). But if, say, all of the featureds were half as likely to show up, that *would* tend to show up here.
The last new champ I pulled was Scarlet Witch, which was the 23rd "new" champ I received from the featured crystals. Statistically speaking, #23 should have arrived on pull #67. It actually arrived on pull #69, which in statistical terms is basically a bullseye. The one champ I never pulled from the featured was Rocket Raccoon. Statistically speaking, I should expect him to show up around crystal #91, or 22 crystals from here (in actuality, I should expect it to take on average 24 crystals from here, because at this point I would be trying to get one out of 24 champs in the crystal, but this opens a different can of statistical analysis worms).
Oh hell no, I'm not opening 24 more featureds to see when Rocket Raccoon shows up.
Sixty nine crystals sounds like a lot, but it actually isn't a statistically significant amount if you are trying to, say, prove that every drop has an equal chance of falling. You'd need tens of thousands of crystals to do that. It is enough to demonstrate that most of the silly conspiracy theories are just that: silly. Here's my overall distribution of drops:

Out of 69 crystals, 22 were featured drops. The statistical average would be about 17 (69/4). So the crystal isn't significantly biased away from the new featured champs. There's not enough data to prove they are *exactly* balanced, but they are statistically unlikely to be biased low. If I should normally get 17 and I actually got 22, that might be lucky. But if someone thinks they are biased in such a way so that featureds come up, say, half as often as they should, then I got 22 when the crystals are rigged to generate something closer to 8. That's very, very unlikely.
69 crystals isn't enough to show that any one particular drop lands statistically at the presumptive percentage, but is it enough data to show *anything* statistically interesting? As it turns out, it is. We can analyze whether the crystals roughly follow gatcha math. What do I mean by that?
Gatcha mechanics generally refers to the situation where a person/player is confronted with an opportunity to get a random reward, selected from a set of rewards, where the presumptive desire is to collect the entire set (or some desirable subset of the set). Now, most people don't actually try to get all 24 champions in the featured crystal (if for no other reason than most people tend to have some of them already), but what if I analyze the data *as if* I was trying to collect them all. Is there enough data to see if the crystal's random drops roughly follows what I would expect? In at least rough terms, it is.
Let's answer one question first. If you were trying to get at least one of all twenty four champions from the crystal, about how long would that take? This isn't math that is often discussed. You might think you could calculate the odds of pulling the first champ, then the odds of pulling the second champ, and so on. But that doesn't work. While trying to pull the fifteenth champ, you might pull the eighteenth champ first, out of order. Then the math vastly overestimates how long it takes. But as it turns out, there is a relatively simple way to calculate this. We look at the average number of pulls it takes before you pull a champ you haven't pulled yet. If we go step by step, the answer drops out naturally.
We start with no champs and no crystals. How many crystals will it take before we pull a champ we haven't pulled yet? Obviously: 1. Whatever is in that crystal, it is guaranteed to be a new pull. Okay, we have one champion. Now how long will it take to pull a new champ? Well, 23 of the 24 champs would be new, and one would be a dup. If it were 12 new champs out of 24, the odds of pulling a new champ would be 50% - 12/24. And the number of crystals we would need to pull, on average, before we got a new champ would be 2, basically the reciprocal of the odds of a new champ. It is pretty obvious when the numbers are easy. But we generalize this for all situations: the odds of pulling a new champ are X/24, where X is 24 minus the number of new champs you have so far. And the average number of crystals you would need to open to get a new champ is the reciprocal of that, or 24/(1-new).
So to get one new champ it would take 24/24 crystals. To get two new champs would take 24/24 + 24/23 crystals. To get three new champs would take 24/24 + 24/23 + 24/22 crystals, and so on. We get this table:

Now these are all my drops:

And this is a table of how many new champs I had, alongside the statistical average expected:

And how close was my distribution to the predicted gatcha new drop rate?

Pretty close. The crystal behaves, over long stretches, about as you would expect if each drop was roughly identical in drop rate. Now, this doesn't *prove* they are all identical in drop rates. It just shows they behave *like* they would. This means certain kinds of "rigging" are unlikely, in particular while it is statistically possible for some champs to be more likely than average, it is not statistically likely that some champs are far less likely than average. More likely champs would show up earlier, which would show up in the data as the blue line starting off higher than the orange line on the left side of the chart. But champs that were deliberately made much rarer would cause the blue line to drop progressively much lower than the orange line as you went to the right. That doesn't appear to be the case.
In other words, if Groot was twice as likely as every other champ, this sort of analysis wouldn't catch that (although the fact that I only pulled Groot twice would strongly contradict that conjecture). But if, say, all of the featureds were half as likely to show up, that *would* tend to show up here.
The last new champ I pulled was Scarlet Witch, which was the 23rd "new" champ I received from the featured crystals. Statistically speaking, #23 should have arrived on pull #67. It actually arrived on pull #69, which in statistical terms is basically a bullseye. The one champ I never pulled from the featured was Rocket Raccoon. Statistically speaking, I should expect him to show up around crystal #91, or 22 crystals from here (in actuality, I should expect it to take on average 24 crystals from here, because at this point I would be trying to get one out of 24 champs in the crystal, but this opens a different can of statistical analysis worms).
Oh hell no, I'm not opening 24 more featureds to see when Rocket Raccoon shows up.
39


Comments
Thanks for putting the time and effort into this. Will be helpful to link it 1-2 times a day every time there is a new tinfoil hat post on a featured crystal.
RIP
That's bait, man.
Og thor and man thing were both sig 20 at the beginning and are now sig 160 each. I still haven’t pulled Valkyrie but I’ve gotten everything else I wanted.
He just didn't pull him from featureds..
Look at your results: you have “clumps” of QS, Titania, Hulkbuster and X-23, while Rocket, MrN, Valk, Thor and OGSW barely appear (or don’t).
Most people understand you are unlikely to get an exactly even distribution. But many don’t get that pRNG will often produce largeish clumps that feel like overweighting or outright error. Add to that the occasional production of crystals with the wrong drop rate and voila—you’ve got a prima facie case of crystal conspiracy.
I stopped sooner than you at #57. Only Star-Lord and Gorr missing from my pulls, but I did manage 7 Valkyries, 6 Rogues, 6 Titanias and 5 Groots. That’s over 40% of my pulls consolidated across just four champions. If you want to add in the 4 Wiccans, then that’s half of my pulls across five champs. Clumpiness indeed.
The point is that across such a small number of opportunities clumpiness rules the day. It’s to be expected. Over thousands and thousands of pulls, clumpiness gets smoothed. But not over 57, which is a lot for me individually but not a lot statistically.
Now, if only the T4c trade-in crystals would stop clumping the opposite way I always need them to…
Dr. Zola
So the point being: opening 69 crystals and not getting a specific champ is statistically what you can expect?!
I also pulled and duped all 6 featured champs except QS. I pulled him but probably won't open more because o want iDoom.
You should unsubscribe from Prof Hoff.
Dr. Zola
But let's say you want two of them. Or three. Or five, or ten. Then what? The odds are always higher at the start, because in effect there are more ways to win. But as you scratch them off your list, the odds of landing one of the rest become smaller and smaller, because in effect your target keeps shrinking. Even the basic crystals obey this mathematical property. The first time anyone opens a 6* basic crystal, the odds are essentially 100% they will get a new champ. But the more champs they get, the harder it is to get new champs.
Psychologically, this can play with people's heads. It can seem like there are no good pulls, when in fact there are tons of good pulls, just pulls a player happens to already have or were not specifically searching for. It has happened more than once that a player will post their "bad pulls" on the forums or the reddit, only to have other players comment on how not bad those pulls actually are. If you're looking for four champs in the crystal, the first three will land far faster than the last one. And because of that, people can get fixated on that last one, regardless of which one it was, and decide the crystal is "rigged" to not give that champ to them. Even though initially, that one champ was no more desirable than the other three. It retroactively becomes the one champ the player wanted that the crystals refuse to give them.
*Something* had to be last. However, many crystals I opened, there would always be a first champ pulled and a last champ I had not pulled yet. This time it was Rocket. But it could have been Quicksilver, or Groot, or Valkyrie, or Winter Soldier, or Galan. When it is Rocket, everyone shrugs and says who cares. When it is Galan, it is a crystal conspiracy.
Player metas are a very powerful homogenizing force. Players who don't know any better follow the leader. They rank up who they are told they should rank up. If everyone got the same drops, 99% of the players would probably have literally identical rosters. Random champion acquisition forces players to try to make due with what they get. It pushes back somewhat on homogenizing metas. If players start off with differing rosters, they might hold onto that deeper into their playing lives knowing they don't have to do what the common meta ideas recommend.
"Clumpy" randomness is one way to look at it: by looking at what players get a lot more of. But "Gappy" randomness is the other way to look at it: focusing on what players don't get precisely because they got more of other things. It is the combination of the clumps and the gaps that give each player a slightly different experience than most other players. This is very, very deliberately baked into why champion acquisition is random.